Lattice energy of MgF2-CaF2-ZrO2 molecule-explanation-calculation

·        Which one has higher lattice energy, MgF₂, CaF₂ or ZrO₂ ?

Lattice energy is directlyproportional to the multiple of ionic charge and inversely proportional to the radius of cation and anion.

In the above compounds, MgF₂, CaF₂ or ZrO₂ , the extent of charge on‘Mg’ ,’Ca’ and ‘Zr’ metal are, +2 ,+2 and +4 respectively.

Again, the extent of charge on ‘F’ and ‘O’ atoms are -1 and -2  respectively.

So, from the data of cationic and anionic charge, it is clear that the multiple of ionic charge for ZrO₂ is highest.

Hence, ZrO₂ molecule has higher lattice energy among all the above compounds.

The value of lattice energy of MgF2, CaF2and ZrO2 molecules are, -2913 Kj/mole , -2609 Kj/mole and  - 8714.5 kJ/ mole respectively.

Read more: Which one has more lattice energy, Na₃N or NaF?

Read more :Calculationof lattice energy of Na3N ?

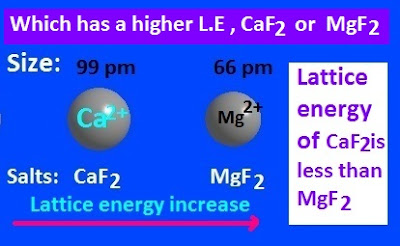
·        Which one has higher lattice energy, MgF₂  or CaF₂ ?

In case of MgF₂ and CaF₂ molecule, the extent of charge on both ‘Mg’ and’ Ca’ metal is, +2 and the extent of charge on ‘F atom is, -1 .

That is, both cations and anions contain same extent of charge.

Now, both calcium and magnesium are group-II alkaline earth metal. It has been found that, the cationic size of group-II metal decreases from top to bottom.

Therefore, the radius of Mg²⁺ cation is less than Ca²⁺ cation.

Since, the lattice energy is directly proportional to the multiple of ionic charge 

and inversely proportional to the radius of cation and anion, hence MgF₂ has higher lattice energy than CaF₂.

The value of lattice energy of MgF2 and CaF2molecules are, -2913 Kj/mole , and -2609 Kj/mole respectively.

·        Why is the lattice energy of ZrO₂ so high ?

Lattice energy is directly proportional to the multiple of ionic charge and inversely proportional to the radius of cation and anion.

Now, in case of ZrO2 molecule, the extent of charge on ‘Zr’ metal atom is very much high, that is, +4 and hence the radius of Zr⁴⁺ cation is small.

Metal with high charge and small radius cause the high lattice energy.

Consequently,  the lattice energy of ZrO₂ so high.

The lattice energy of ZrO₂ molecule is about, –9714.5 Kj/mole.

Read more: Which one has more lattice energy, Na₃N or NaF?

Read more :Calculationof lattice energy of Na3N ?

·        Lattice energy calculation for ZrO₂ molecule

Lattice energy of ZrO₂ can be calculated indirectly by using Born-Haber cycle. 

The atomic number of oxygen and zirconium are 8 and 40 respectively.

Hence, the electronic configuration of  ‘O’ and ‘Zr’ are  [He]2s² 2p⁴ and [Kr] 4d² 5s² respectively.

The first and second electron affinities of oxygen atom is +141 kj/mole and – 770 kj/mole respectively. The bond dissociation energy of oxygen molecule is 498 kj/ mole.

The sublimation energy of Zr(s ) to Zr(g) change is 591 kj/mole and first, second , 

third and forth ionization energy of Zr are about 640 kj/mole, 1270 kj/mole, 2218 kj/mole and 3412 kj/mole respectively.

Applying, the above data, we can calculate the lattice energy of ZrO₂ molecule. ΔH_f  of ZrO₂ molecule is  –1080 kj/mole. From Born-Haber cycle,

ΔH_f = S + [ I.E₁ + I.E₂ + I.E₃ + I.E₄ ] + D + 2 x [ E.A₁ + E.A₂ ] + U

or,  –1080  = 591 + [ 640 +1270 +2218 +3412] + 2 x [ +141–770]  +U

or,  –1080  = 591 + 7540 + 2 x (–629)  + U

or,  –1080  = 591 + 7540 – 1258   +  U

Therefore,  U  = –591 – 7540 + 1258 – 1080  =  – 8451 kj/mole.

That is, the lattice energy of ZrO₂ is –8451 kj/mole.

      ·        Which one has higher lattice energy, MgF₂, CaF₂ or ZrO₂ ?

·        Which one has higher lattice energy, MgF2  or CaF2 ?

·        Why is the lattice energy of ZrO₂ so high ?

·        Lattice energy calculation for ZrO₂ molecule

·        What is lattice energy ?

·        What is unit of lattice energy ?

·        How lattice energy affect boiling point ?

·        How lattice energy influence melting point ?

Lattice energy and Born-Lande equation.